mathematics standards to grade 5

Standard 1 — Number Sense
Understanding the number system is the basis of mathematics. Students extend their understanding of the magnitudes of numbers to rounding whole numbers and decimals to any place value. They order and compare whole numbers and decimals using the correct symbols for greater than and less than. They develop the concept of percentage as parts of a hundred and compare different ways of looking at fractions. They identify whole numbers as prime or composite, and they compare fractions, decimals, and mixed numbers on a number line.

Standard 2 — Computation
Fluency in computation is essential. Students extend the standard methods for multiplying and dividing to larger numbers. They add and subtract more complex fractions and decimals, learning how these different representations of numbers can be manipulated. They also develop an understanding of how to multiply and divide fractions.

Standard 3 — Algebra and Functions
Algebra is a language of patterns, rules, and symbols. Students at this level develop further the fundamental concept of a variable — having a letter stand for all numbers of a certain kind. They use this to write simple algebraic expressions and to evaluate them. They begin to develop the idea of linking an algebraic equation to a graph, by finding ordered pairs that fit a linear equation, plotting these as points on a grid, and drawing the resulting straight line. They also interpret graphs to answer questions.

Standard 4 — Geometry
Students learn about geometric shapes and develop a sense of space. They draw angles, parallel and perpendicular lines, the radius and diameter of circles, and other geometric shapes, using ruler, compass, protractor, and computer drawing programs. They identify congruent triangles and explain their reasoning using specific geometrical terms , such as equilateral, isosceles, acute, and obtuse. They classify polygons with five or more sides. They develop an understanding of reflectional and rotational symmetry, and they construct prisms and pyramids, developing their ability to work in three dimensions.

Standard 5 — Measurement
The study of measurement is essential because of its uses in many aspects of everyday life. Students develop and use the formulas for calculating perimeters and areas of triangles, parallelograms, and trapezoids. They extend these ideas to finding the volume and surface area of rectangular solids. They understand and use additional units for measuring weight: ounce, gram, and ton. They also add and subtract with money in decimal notation.

Standard 6 — Data Analysis and Probability
Data are all around us — in newspapers and magazines, in television news and commercials, in quality control for manufacturing — and students need to learn how to understand data. At this level, they use the mean, median, mode, and range to describe data sets. They further develop the concept of probability, recording probabilities as fractions between 0 and 1 and linking these to levels of certainty about the events described.

Standard 7 — Problem Solving
In a general sense, mathematics is problem solving. In all of their mathematics, students use problem-solving skills: they choose how to approach a problem, they explain their reasoning, and they check their results. As they develop their skills with algebra, geometry, or measurement, for example, students move from simple to more complex ideas by taking logical steps that build a better understanding of mathematics.

As part of their instruction and assessment, students should also develop the following learning skills by Grade 12 that are woven throughout the mathematics standards:

Communication
The ability to read, write, listen, ask questions, think, and communicate about math will develop and deepen students’understanding of mathematical concepts. Students should read text, data, tables, and graphs with comprehension and understanding. Their writing should be detailed and coherent, and they should use correct mathematical vocabulary. Students should write to explain answers, justify mathematical reasoning, and describe problem-solving strategies.

Reasoning and Proof
Mathematics is developed by using known ideas and concepts to develop others. Repeated addition becomes multiplication. Multiplication of numbers less than ten can be extended to numbers less than one hundred and then to the entire number system. Knowing how to find the area of a right triangle extends to all right triangles. Extending patterns, finding even numbers, developing formulas, and proving the Pythagorean Theorem are all examples of mathematical reasoning. Students should learn to observe, generalize, make assumptions from known information, and test their assumptions.

Representation
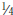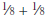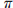The language of mathematics is expressed in words, symbols, formulas, equations, graphs, and data displays. The concept of one-fourth may be described as a quarter, , one divided by four, 0.25, , 25 percent, or an appropriately shaded portion of a pie graph. Higher-level mathematics involves the use of more powerful representations: exponents , logarithms, , unknowns, statistical representation, algebraic and geometric expressions. Mathematical operations are expressed as representations: +, =, divide, square. Representations are dynamic tools for solving problems and communicating and expressing mathematical ideas and concepts.

Connections
Connecting mathematical concepts includes linking new ideas to related ideas learned previously , helping students to see mathematics as a unified body of knowledge whose concepts build upon each other. Major emphasis should be given to ideas and concepts across mathematical content areas that help students see that mathematics is a web of closely connected ideas (algebra, geometry, the entire number system). Mathematics is also the common language of many other disciplines (science, technology, finance, social science, geography) and students should learn mathematical concepts used in those disciplines. Finally, students should connect their mathematical learning to appropriate real-world contexts.

Standard 1

Number Sense

Students compute with whole numbers*, decimals, and fractions and understand the relationship among decimals, fractions, and percents. They understand the relative magnitudes of numbers. They understand prime* and composite* numbers.

5.1.1
Convert between numbers in words and numbers in figures, for numbers up to millions and decimals to thousandths.
Example: Write the number 198.536 in words.

5.1.2
Round whole numbers and decimals to any place value.
Example: Is 7,683,559 closer to 7,600,000 or 7,700,000? Explain your answer.

5.1.3
Arrange in numerical order and compare whole numbers or decimals to two decimal places by using the symbols for less than (<), equals (=), and greater than (>).
Example: Write from smallest to largest: 0.5, 0.26, 0.08.

5.1.4
Interpret percents as a part of a hundred. Find decimal and percent equivalents for common fractions and explain why they represent the same value.
Example: Shade a 100-square grid to show 30%. What fraction is this?

5.1.5
Explain different interpretations of fractions: as parts of a whole, parts of a set, and division of whole numbers by whole numbers.
Example: What fraction of a pizza will each person get when 3 pizzas are divided equally among 5 people?

5.1.6
Describe and identify prime and composite numbers.
Example: Which of the following numbers are prime: 3, 7, 12, 17, 18? Justify your choices.

5.1.7
Identify on a number line the relative position of simple positive fractions, positive mixed numbers, and positive decimals.
Example: Find the positions on a number line of 1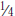 and 1.4.

* whole number: 0, 1, 2, 3, etc.
* prime number: a number that can be evenly divided only by 1 and itself (e.g., 2, 3, 5, 7, 11)
* composite number: a number that is not a prime number (e.g., 4, 6, 8, 9, 10)

Standard 2

Computation

Students solve problems involving multiplication and division of whole numbers and solve problems involving addition, subtraction, and simple multiplication and division of fractions and decimals.

5.2.1
Solve problems involving multiplication and division of any whole numbers.
Example: 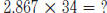. Explain your method.

5.2.2
Add and subtract fractions (including mixed numbers) with different denominators .
Example: .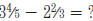

5.2.3
Use models to show an understanding of multiplication and division of fractions.
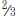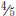Example: Draw a rectangle 5 squares wide and 3 squares high. Shade of the rectangle, starting from the left. Shade of the rectangle, starting from the top. Look at the fraction of the squares that you have double -shaded and use that to show how to multipl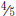y by .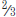

5.2.4
Multiply and divide fractions to solve problems.
Example: You have 3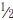 pizzas left over from a party. How many people can have 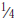of a pizza each?

5.2.5
Add and subtract decimals and verify the reasonableness of the results.
Example: Compute 39.46 -20.89 and check the answer by estimating.

5.2.6
Use estimation to decide whether answers are reasonable in addition, subtraction, multiplication, and division problems.
Example: Your friend says that 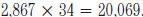. Without solving, explain why you think the answer is wrong.

5.2.7
Use mental arithmetic to add or subtract simple decimals.
Example: Add 0.006 to 0.027 without using pencil and paper.

Standard 3

Algebra and Functions

Students use variables in simple expressions, compute the value of an expression for specific values of the variable, and plot and interpret the results. They use two-dimensional coordinate grids to represent points and graph lines.

5.3.1
Use a variable to represent an unknown number.
Example: When a certain number is multiplied by 3 and then 5 is added, the result is 29. Let x stand for the unknown number and write an equation for the relationship.

5.3.2
Write simple algebraic expressions in one or two variables and evaluate them by substitution.
Example: Find the value of 5x +2 when x t =3.

5.3.3
Use the distributive property* in numerical equations and expressions.
Example: Explain how you know that .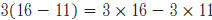

5.3.4
Identify and graph ordered pairs of positive numbers.
Example: Plot the points (3, 1), (6, 2), and (9, 3). What do you notice?

5.3.5
Find ordered pairs (positive numbers only) that fit a linear equation , graph the ordered pairs, and draw the line they determine.
Example: For x = 1, 2, 3, and 4, find points that fit the equation y =2x + 1. Plot those points on graph paper and join them with a straight line.

5.3.6
Understand that the length of a horizontal line segment on a coordinate plane equals the difference between the x-coordinates and that the length of a vertical line segment on a coordinate plane equals the difference between the y-coordinates.
Example: Find the distance between the points (2, 5) and (7, 5) and the distance between the points (2, 1) and (2, 5).

5.3.7
Use information taken from a graph or equation to answer questions about a problem situation.
Example: The speed (v feet per second) of a car t seconds after it starts is given by the formula v = 12t. Find the car’s speed after 5 seconds.

* distributive property: e.g.,